How high is your frustum?
Sometimes working on the maths describing something very concrete – something you can picture in your head – can lead you to stumble upon maths that you might not ever have imagined.
Let's go back the first century AD, when the Greek mathematician Heron was contemplating a frustum – a square based pyramid with the top lopped off. If the length of the sides of the square base is a, the length of the sides of the square top is b, and the length of the sloping edges running from top to bottom is c, how high is your frustum?
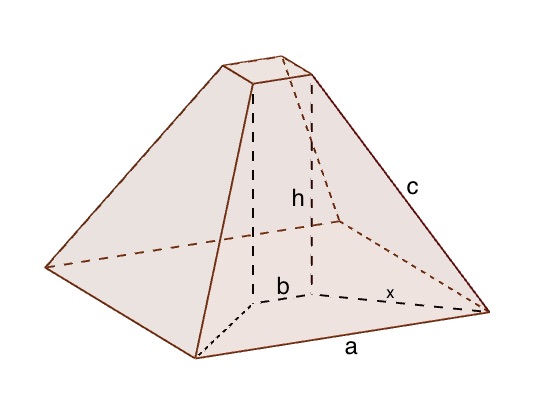
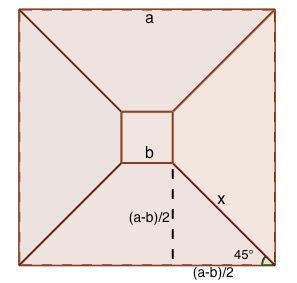
In the picture above the lengths of the sides and height are labelled as we've described. To the right is the view from the top of the frustum. In this view the square top is projected onto the square base, making a trapezoid with base length 
, top length 
, and side length 
. The base of the trapezoid is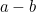 
longer than the top. Consider the right-angled triangle on each side of the trapezoid, formed by the its height, half the extra base length, 
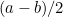, and the side of length . The angle to the base of the trapezoid is 45 degrees (as it is half of a right angle), and so the angles, and sides, either side of the right angle in this triangle are equal. This means the height of the trapezoid is also 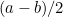. And so using Pythagoras' theorem we get
![\[ x=\sqrt{2 \left( \frac{(a-b)}{2} \right) ^2}=\frac{(a-b)}{\sqrt2}. \]](/MI/f7fe86d810b8f1d561bdec1fd42f12c9/images/img-0006.png) |
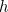, base and hypotenuse 
. Using Pythagoras' theorem again we get: ![\[ h = \sqrt{c^2 - \frac{(a-b)^2}{2}}. \]](/MI/f7fe86d810b8f1d561bdec1fd42f12c9/images/img-0009.png) |
Heron published this formula for the height of a frustum in his book Stereometria and demonstrated it with the values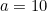 
, 
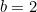and 
. The picture above is of a frustum with these measurements, and agrees with the formula that the height would be 
.
He went on to try his formula with a different example, with values of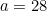 
, 
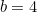and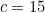 
. Plugging these values into the formula gives the height of such a frustum to be
![\[ h= \sqrt{225 - 288} = \sqrt{-63}. \]](/MI/0ec704d8db8cab15ffb42551211e4a35/images/img-0004.png) |
Heron had come up with an impossible example. And he'd also given us the first recorded example of a calculation involving the square root of a negative number – the first step on our journey to discovering imaginary and complex numbers.
But it was centuries before anyone would even contemplate such strange mathematical beasts, and so Heron fudged his working and recorded the answer as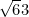 
. One mathematical step at a time!
Rachel Thomas and Marianne Freiberger are the editors of Plus. They came across this story while researching their book Numericon: A journey through the hidden lives of numbers.